
Continuous data result from infinitely many possible values that correspond to some continuous scale that covers a range of values without gaps, interruptions, or jumps.

the test statistic needed to test this claim= 10.92Ĭritical value ( one-tailed) for 0.01 significance level =ĭecision : Since Test statistic value (10.92)> Critical value (2.326), so we reject the null hypothesis. In a test of a method of gender selection developed by the Genetics & IVF Institute, 726 couples used the XSORT method and 668 of them had girls. Preliminary results from a test of the XSORT method of gender selection involved 14 couples who gave birth to 13 girls and 1 boy. for a hypothesis test of the claim that when parents use a particular method of gender selection, the proportion of baby girls is different from 0.5. Test statistic :, where n is sample size, is sample proportion and p is the population proportion. In a test of the XSORT method of gender selection, 574 babies are born to couples trying to have baby girls, and 525 of those babies are girls (based on data from the Genetics \& IVF Institute).

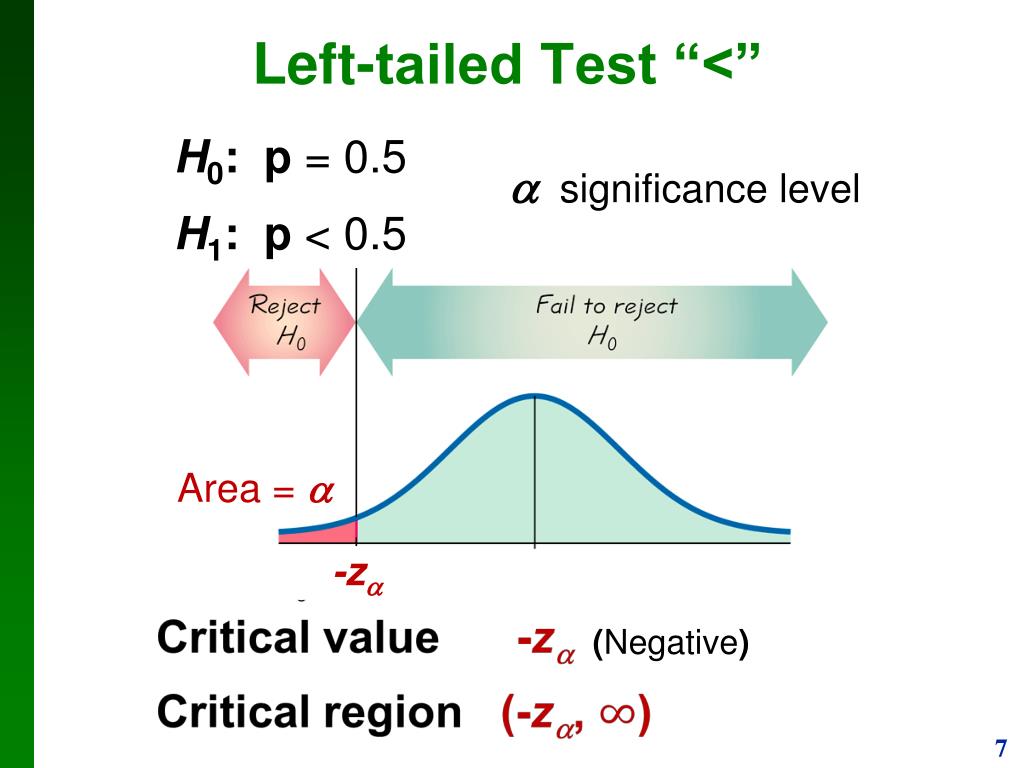
Testing the connection to the Tivoli Data Warehouse database is valid even with an. Use the given claim and the preliminary results to calculate the value of the test statistic. Gender Selection In updated results from a test of MicroSort’s XSORT gender-selection technique, 726 births consisted of 668 baby girls and 58 baby boys (based on data from the Genetics & IVF Institute). A monitoring process fails to start on Linux or UNIX after changing a. use the XSORT method of gender selection and each couple gives birth to one baby. Preliminary results from a test of the XSORT method of gender selection involved 100 couples who gave birth to 58 girls and 42 boys. Therefore, we would say that that that technique does not seem to be. Since is right-tailed, so the hypothesis test is a right-tailed z-test.Īlso, it is given that, the sample size : n= 291 Example Lets again consider the claim that the XSORT gender selection method increases the likelihood of having a girl. I.e The population proportion of giving birth to a boy = 0.5 We know that the probability of giving birth to a boy : p= 0.5
#In the test of the xsort method of gender selection free
Click here to view all solutions)/RD/Rect/Subj(Typewritten Text)/Subtype/FreeText/T(Bully)/Type/Annot>endobj16945 0 obj/BS 16948 0 R/Contents(Click ads to keep site free of charge)/CreationDate(D:20111102153241-07'00')/DA(11 TL /Cour 8 Tf)/DS(font: Times 8.0pt text-align:left color:#000000 )/F 4/IT/FreeTextTypewriter/M(D:20111102203121-07'00')/NM(69d391a7-93b6-43a7-92b7-121fd6524c56)/P 2185 0 R/RC(Ĭlick ads to keep site free of charge)/RD/Rect/Subj(Typewritten Text)/Subtype/FreeText/T(Bully)/Type/Annot>endobj16946 0 obj/Border/H/N/Rect/Subtype/Link/Type/Annot>endobj16947 0 objendobj16948 0 objendobj16949 0 obj/ProcSet>/Subtype/Form/Type/XObject>stream Finding the Value of the Test Statistic Lets again consider the claim that the XSORT method of gender selection increases the likelihood of having a baby.


 0 kommentar(er)
0 kommentar(er)
